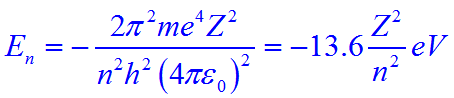
Watch the following video to get an idea about Energy of electron in Bohr's orbit.
(IIT JEE 1998)
a) -3.4 eV
b) -4.2 eV
c) -6.8 eV
d) +6.8 eV
Answer: a
Logic:
The energy of an electron in Bohr’s orbit of hydrogen atom is given by the expression:
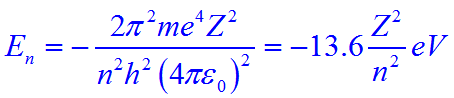
Since Z = 1 for hydrogen above equation can be further simplified to:
En = -13.6/n2 eV
The energies of electrons in the Bohr's orbits of hydrogen atom expressed in eV are:
| Orbit | Energy |
| 1 | -13.6/12 = -13.6 eV |
| 2 | -13.6/22 = -3.4 eV |
| 3 | -13.6/32 = -1.51 eV |
| 4 | -13.6/42 = -0.85 eV |
Excited state(s) represent n = 2, 3, 4 ...... (greater than 1).
Note:
The ratio of energy of electrons in the orbits of hydrogen atom is:
E1 : E2 : E3 : E4 ........... = 1/12 : 1/22 : 1/32 : 1/42 .......... = 1 : 1/4 : 1/9 : 1/16 ..........
Conclusion:
Correct option is "a".
a) Increases
b) Decreases
c) Remains constant
d) Decreases for lower values of n and increases for higher values of n
Answer: b
Solution:
From the previous problem, we can clearly see the decrease in energy difference between adjacent levels with increase in the principal quantum number, n. (see the table or ratio)
a) -e2/r
b) -e2/r2
c) -e2/2r
d) -e2/2r2
Answer: c
Solution:
Total Energy of electron, Etotal = Potential energy (PE) + Kinetic energy (KE)
For an electron revolving in a circular orbit of radius, r around a nucleus with Z positive charge,
PE = -Ze2/r
KE = Ze2/2r
Hence:
Etotal = (-Ze2/r) + (Ze2/2r) = -Ze2/2r
And for H atom, Z = 1
Therefore:
Etotal = -e2/2r
Note:
This is not a good question because 'r' value is a variable and depends on the principal quantum number, n. Actually it is the energy of electron in the nth orbit and not just for 1st orbit.
Then why this question is added?
To show that the questions given in entrance exams are always not perfect. So think outside the box too.
What to do when questions like this are asked?
Be flexible in your thinking. Though questions like this are not perfect, choose the correct answers wisely among the options given.
a) 8.51 x 105 J mol-1
b) 6.56 x 105 J mol-1
c) 7.56 x 105 J mol-1
d) 9.84 x 105 J mol-1
Answer: d
Logic:
We know that energy of nth orbit,
En = -K/n2 (for hydrogen atom), where K is a constant.
The energy required to excite the electron from n = 1 to n = 2 is:
ΔE(2,1) = E2- E1
= (-K/n22) - (-K/n12)
= K(1/n12 - 1/n22)
= K(1/12 - 1/22)
So we have to know the value of 'K'. This can be done by using ionization enthalpy data.
Ionization enthalpy is the energy required to take the electron from n = 1 orbit to n = ∞ orbit. Hence ionization energy must be equal to the energy difference between these two orbits.
i.e.
Ionization energy = ΔE(∞,1) = E∞- E1
ΔE(∞,1) = (-K/n∞2) - (-K/n12) = (-K/∞2) - (-K/12) = K/n12 = K
Therefore:
K = 1.312 x 10-6 J mol-1
Solution:
Now we can calculate the energy required to excite the electron from n = 1 to n = 2 as follows.
ΔE(2,1) = K(1/12 - 1/22)
= 1.312 x 10-6 J mol-1 (1/12 - 1/22)
= 9.84 x 105 J mol-1
Note:
Ionization energy = ΔE(∞,1) = E∞- E1 = - E1
Hence we can take negative of ionization energy as the energy of the ground state (n=1).
1) 4.41 x 10-16 J atom-1
2) -4.41 x 10-17 J atom-1
3) -2.2 x 10-15 J atom-1
4) 88.2 x 10-17 J atom-1
Answer: 2
Logic:
Since negative of Ionization energy is the energy of first stationery state, for He+, the energy of 1st level is -19.6 x 10-18 J atom-1.
The energy of electron in 1st level for He+ can be written as:
E1 = -K(Z2/n2) = -K x (22/12) = -4K
Hence
K = -19.6 x 10-18 / 4 = 4.9 x 10-18 J atom-1
Solution:
The energy of first energy level of Li2+ = -K(Z2/n2) = -K x (32/12) = -9K = -9 x 4.9 x 10-18 = -4.41 x 10-17 J atom-1
1) 1.216 x 10-7m
b) 2.816 x 10-7m
c) 6.500 x 10-7m
d) 8.500 x 10-7m
Answer: 1
Logic:
Energy required to excite electron from n=1 to n=2 will be equal to the energy difference between these levels.
i.e.
ΔE(2,1) = E2- E1
= (-K/n22) - (-K/n12) (for H atom, Z = 1)
= K(1/n12 - 1/n22)
= K(1/12 - 1/22)
= 3K/4
= (3/4) x 2.178 x 10-18 J
= 1.6335 x 10-18 J
Solution:
The wavelength corresponding to above excitation:
λ = hc/E = (6.626 x 10-34 J s x 3.0 x 108 m s-1) / (1.6335 x 10-18 J )
= 1.216 x 10-7m
(IIT JEE ADVANCED - 2013 )
a) Larger the value of n, the larger is the radius of orbit.
b) Equation can be used to calculate the change in energy when the electron changes orbit.
c) For n=1, the electron has a more negative energy than it does n=6 which means that the electron is more loosely bound in the smallest allowed orbit.
d) The negative sign in equation simply means that the energy for electron bound to the nucleus is lower than it would be if the electrons were at the infinite distance from the nucleus.
Answer:
option "c" is the wrong statement.
Explanation:
The energy of electron in a particular orbit is equal to the loss in energy of electron when it is taken from infinite orbit to that orbit. Energy of electron in the infinite orbit is zero which also indicates there is no attraction between nucleus and electron. But when it is bring closer towards the nucleus, there is loss of energy due to attraction and hence the energy in the orbitals for which n < ∞ is always negative. Greater the negative value greater is the attraction.
Hence the last half part of statement given in the option "c" is wrong.
1) 1
2) 25
3) 5
4) 36
Answer: 2
Solution:
First of all we have to find the n value for the energy level.
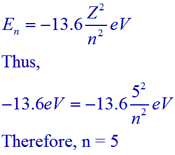
For one electron atomic system (hydrogen like atom), the orbitals in a given orbit are degenerate irrespective of their azimuthal quantum number (l). The energy is decided by principal quantum number (n) only. Hence the number of degenerate orbitals is equal to the number of orbitals in a principal quantum level and is given by n2.
Therefore, the degeneracy for the 5th level in hydrogen like atom (or ion) = 52 = 25.
(new) Click here to see 3d Interactive Solved Question paper
1) Write the values of energy of ground state in hydrogen atom in different units.
2) What is the ratio of energies of electrons in the ground states of H, He+, Li2+and Be3+?
3) Calculate the atomic number of hydrogen like species which can be ionized by an electron moving with a velocity, v = 6.56 × 106 m s-1 .
Hint: The electron must be in the first orbit, since it is hydrogen like species i.e. n = 1. Now we have to find the atomic number, Z from the equation
v = 2.18 x 106 x Z m s-1
Z = v / 2.18 x 106 x Z m s-1 = 6.56 × 106 m s-1 / 2.18 x 106 x Z m s-1= 3
The species is Be2+.
Click here for more solved problems on velocity of electrons.
4) Write the formula/expression for energy of electron in the nth orbit of hydrogen atom.
5) What is the kinetic energy of nth orbit of hydrogen atom.
6) How do you calculate the total energy of electron in the nth stationary orbit of hydrogen atom?
7) The energy of an electron in the nth Bohr's orbit is proportional to ____________ .
Question- 8) What is the energy of electron in 3rd Bohr's orbit of hydrogen atom?
Answer: -1.51 eV
Question-9) Kinetic energy (KE) of electron in a particular orbit is 3.4 eV. The potential energy is ___________ .
Answer: -6.8 eV
10) If energy of electron in a hydrogen atom is -RH /9. The possible number of orbitals in this hydrogen atom is _______ . Where RH is Rydberg constant.
Question-11) What is the difference in the energies of 1st and 2nd Bohr's orbits of H-atom?
Answer: -3.4 - (-13.6) = 10.2 eV
Question-12) What is the total energy of an electron in the n=4 Bohr orbit of the hydrogen atom ?
Answer: -0.85 eV.
13) Calculate the energy required to excite an electron of Hydrogen atom from first orbit to second orbit.
Question-14) Why is the energy of electron negative in the hydrogen atom?
Answer: The energy of free electron (when there is no attraction with nucleus) is arbitrarily fixed as zero and the energy decreases when it is attracted towards nucleus.
15) What is the potential energy of an electron present in n shell of Be3+ ion?
Question-16) What is the energy possessed by an electron for n=infinity?
Answer: Arbitrarily fixed as zero. Not that it should be free from any other external forces of attraction or repulsion as well as it should possess zero velocity.
17) Write the ratio of energy of the electron in ground state of hydrogen.
Question-18) Calculate the potential energy of an electron in the first Bohr orbit of Li2+ ion?
Answer: 122.4 eV
19) The energy of an electron in the nth Bohr orbit of hydrogen atom is given by ......
20) What is the kinetic energy of electron revolving in second excited state?
Question-21) What minimum amount of energy (in J) is required to bring an electron from ground state of Be3+ ion to infinity?
Answer: 217.6 eV
Question-22) If the energy of electron in 1st orbit of hydrogen is –13.6 ev/atom then the energy of electron in 4th orbit of He+ ion is (in ev/atom)..........
Answer: -13.6 eV
23) The energy required to remove an electron in a hydrogen atom from n=10 state is ...........
Question-24) If the energy of third orbit of He+ ion is –x kJ mol–1, then the energy of second orbit of H-atom (in kJ mol–1) will be......
Answer: -9x/16 kJ mol-1
Hint: E(H in 2nd orbit) : E(He in 3rd orbit) = (Z/n)2H : (Z/n)2He = (1/2)2 : (2/3)2 = 9:16
25) The ratio of the energy of electrons in 1st shell of He+ and 3rd shell of Li+2 is.............
26) Potential energy of electron in second orbit of Li2+ is ..........
27) If the ionization potential of hydrogen atom is 13.6ev, then the energy required to remove the electron from the third orbit of hydrogen atom is nearly in ev is ..........
Question-28) Relation between potential energy, kinetic energy and total energy of an electron in a particular orbit is given by.........
Answer: PE = -2KE = 2TE
29) Calculate the energy required to send an electron of Li+2 ion from ground state to 2nd excited state in J/mol.
30) The energy of second orbit of hydrogen is equal to the energy of ..............
Question-31) The ratio of the kinetic energy and the potential energy of electron in the hydrogen atom will be ..........
Answer: 1:(-2)